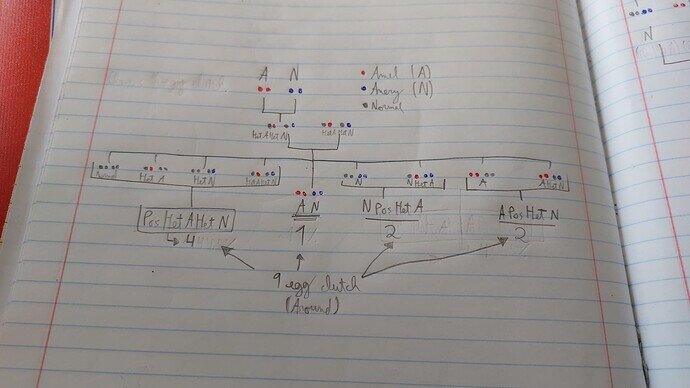
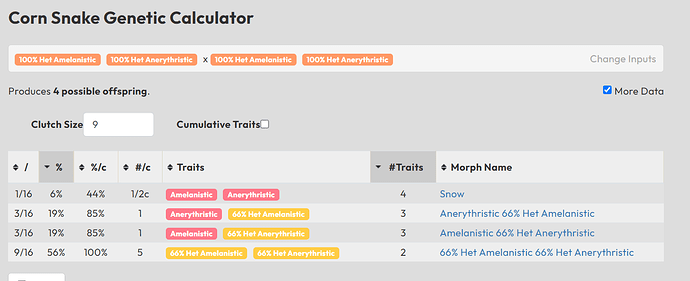
Hey hows it going? Just wanted some clarification here to like what did I miss in this genetics because what I did is almost completly off with the actual calculation.
I’m having a bit of a hard time reading your chart. People typically use a punnett square to calculate genetic odds if they’re going to do it by hand. Are you breeding a het anery, amel to another het anery, amel?
4 Likes
Yes, having 2 red dots means Homozygous Amel. One gray and one red means Het Amel
1 Like
It looks like the calculator is giving you the same results as what you calculated by hand. The babies you should expect are normals 66% poss het amel and anery, amels 66% poss het anery, aneries 66% poss het amel, and snows. The odds are for each individual egg. So the odds for getting a snow is 1/16 for each egg. The odds of getting an anery or an amel will be 3/16 for each egg. The odds of getting a normal will be 9/16 for each egg. Is that what you’re asking? If not, feel free to correct me.
3 Likes
From what I’m seeing, you are getting the same results. I think you may be confused by results being presented in two different ways. What is it that you think differs?
One more note: Very rarely does an actual, real live clutch precisely mirror the statistical likelihoods. YMMV for sure!
2 Likes
Oh thank you, I was asking because in the calculator, I put a 9 egg clutch, and it gave me 5 Pos Het Amel Het Anery, which in my chart was 4 babies in 9 eggs. Then in the calculator, 1 Amel pos het amel, in my it was 2. Same for the anery. And also in my chart I should get a Snow every 9 eggs
1 Like
I’m not a computer programmer, but if I were to guess, the difference between your result and the calculator is due to fractions. The calculator gives results as a percentage likelihood. This calculation yields some decimal results. Since obviously each egg hatches a complete animal, those fractions are rounded.
True - but only in your chart. It’s perfectly possible that you get seven snows in your clutch of nine. It’s also possible that you get none. Odds are odds, not absolutes nor requirements. Mother Nature and Mr. Murphy don’t give a purple fig for our odds calculations. 
3 Likes
Ok then. Anyways I should better rely on the calculator aha
2 Likes
That’s what I do. I can do the calculations on paper, but the computer is a whole lot faster! 
I’m curious, are you doing this pairing this season?
2 Likes
Sorry for just answering now, this pairing was just a test to see if I understood genetics. Im doing something more complex in a few seasons. Objective is a Tessera Xanthic and eventually Tessera Citrine.
Sounds pretty. I’m partial to Caramel- based morphs (among other things). I am expecting a prelay shed for a first clutch soon from a likely Citrine girl het stripe. She is Xanthic Snow minimum but always had a bit of something different. This clutch will prove which she is.
1 Like
Yeah! Mystery babies!
1 Like
You are missing some outcomes. I’m trying to show you, why you are wrong with “one of nine is a snow”. It is in fact “one of sixteen”.:
= normal
= Amel
= Anery
For example you write for het Amel but you forget is a possibility, too. This sounds like it’s not important, but in fact it is important. When you add all the missing outcomes, you will see 16 possibilities:
= normal, no hets
= normal het Amel (from mother)
= normal het Amel (from father)
= Amel, no hets
= normal het Anery (from mother)
= normal het Anery (from father)
= Anery, no hets
= normal het Amel, Anery (both from mother)
= normal het Amel (from father), Anery (from mother)
= normal het Amel (from mother), Anery (from father)
= normal het Amel, Anery (both from mother)
= Amel het Anery (from mother)
= Amel het Anery (from father)
= Anery het Amel (from mother)
= Anery het Amel (from father)
= Snow
So you will get: 9x normal; 3x Amel; 3x Anery; 1x Snow
All with 66% poss het Amel or Anery or both, because 2 of 3 are het and you can’t see which ones are het and which not.
Cheers
Seriva
6 Likes
Nice graphics, Seriva! 
In the end, of course, any individual clutch can vary wildly from correctly predicted outcomes.Statistical probability is valuable for planning, and likely to happen over a large number of eggs, but it’s certainly not a true template.
5 Likes
Yes, and I always like to point out that the probabilities are per egg, not per clutch. So if you have a clutch of 16 eggs and you are hoping for a morph that has a 1/16 chance, you’re not guaranteed to get one egg with that morph. Each egg in the clutch has a 1/16 chance to be that morph.
5 Likes
That is exactly the point that most miss. Thanks!
4 Likes
Oh thats so right! Thank you so much for explaining, now it makes sence
1 Like